How To Find Mass Using Density And Volume

The tube is bent into a "U" shape and mounted on an inexpensive piezoelectric speaker. The resonance frequency of the tube is detected by a photointerrupter, amplified, and fed into the speaker; the resulting feedback circuit keeps the glass tube vibrating at its resonance frequency. A peristaltic pump and computer are used to flow samples through the tube and record the resonance frequency of the tube. Detail of the glass tube shows the path followed by a sample inside the tube as it flows into the sensor ("a"), passes the tip of the sensor ("b"), and exits the sensor ("c").

Resonance frequency of the vibrating tube vs. time as a 770 μm diameter glass bead is passed back and forth through the tube eighteen times. Each passage of the bead through the tube results in a momentary decrease in the tube's resonance frequency; this is recorded as a downward peak in the resonance frequency . Each point on the peak (baseline "a," tip "b," and baseline "c") corresponds with the bead's location in above. The height of this peak is used to determine the buoyant mass of the bead . Histograms showing the buoyant mass of another bead weighed thousands of times in two different fluid densities.

In deionized water (density 1.000 g/mL) the bead has an average buoyant mass of 3.69 ±0.12 μg, and in a sodium chloride solution (density 1.046 g/mL) the bead has an average buoyant mass of −4.42 ±0.16 μg. The widths of these distributions—120 and 160 nanograms—provide an estimate of the resolution of our mass measurements. Before using a vibrating tube to weigh a sample, the tube must first be calibrated. We calibrated the sensor shown in Fig 1A using a glass bead of known size and density in a fluid of known density . Fig 1C shows eighteen downward peaks in the resonance frequency of the tube as the bead is pumped back and forth eighteen times through the tube. The height of each peak (72 millihertz; Fig 1D) is proportional to the buoyant mass of the bead .

From this relationship we obtain the calibration constant c2 for this tube sensor in micrograms per millihertz (4.8 μg/mHz) that can subsequently be used to determine the buoyant mass of any object flowing through this tube. Our homemade vibrating tube mass sensors typically have quality factors around 500, which is comparable to that of tuning forks and high enough for precise measurement of the tube's resonance frequency . The four proof-of-concept samples studied here—microbeads, embryos, seeds, and biomaterials—are representative of a wide range of samples that may be analyzed in fluid using vibrating glass tube sensors.

Our technique is very versatile because all objects have fundamental physical properties like mass. Consequently, our mass sensor can be applied to problems as diverse as screening toxic substances, understanding the growth of plants, measuring the degradation of biomaterials, and many others. And unlike imaging-based measurements of size, our mass sensor is insensitive to the shape of the object. Finally, the automation, portability, and low cost of this technique make vibrating glass tubes particularly well suited for applications in the field or in resource-limited settings. The ideal density measurement would directly monitor the mass and volume of each cell within a population with minimal sample perturbation and allow cells to be collected for subsequent measurements.

Toward this aim, we utilized the suspended microchannel resonator to measure single-cell buoyant mass with high precision. The SMR is a silicon cantilever with an embedded microfluidic channel that resonates at a frequency proportional to its total mass, which changes as individual cells flow through the channel (Fig. 1) . Unlike density gradient centrifugation, this technique provides buoyant mass and volume information, measures growth-arrested cells in almost any medium, and does not require density gradient chemicals. With this method we found that cell density increases prior to bud formation at the G1/S transition. To investigate the origin of this density increase, we used the SMR in a second method where relative density changes of growing yeast cells are measured as the cells are sampled by the microchannel.

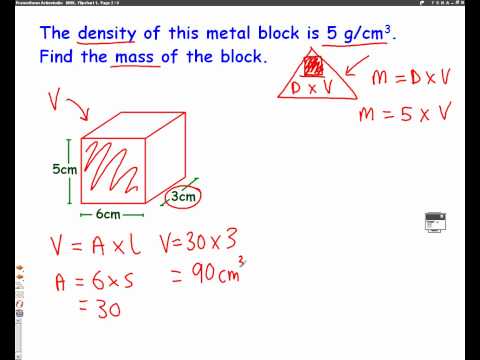
How To Find Mass With Density And Volume Example We found that the density increase requires energy, passage through START, function of the protein synthesis regulator target of rapamycin , and an intact actin cytoskeleton. In conjunction with these density measurements, FACS analysis and bud emergence data suggest that this density change is independent of DNA replication and may result from several START-dependent events. In this work, we demonstrate a simple and inexpensive sensor capable of weighing microgram-sized objects in fluid.

Like the SMR, this sensor uses a change in resonance frequency to weigh an object in fluid with high precision. But unlike the SMR, this sensor can weigh samples with a large range of sizes and is extremely simple to fabricate. Our sensor consists of a short length of glass tubing bent into a "U" shape and attached to an inexpensive speaker that vibrates the glass tubing at its resonance frequency. The resulting sensor shown in Fig 1 costs about US $12 in materials and can be made in under 10 minutes. Additionally, by weighing samples in fluids of different densities, we can also use our sensor to measure the volume and density of samples in fluid.

The SMR reads out real-time changes in relative cell density via the direction of change in buoyant mass as synchronized and growing cells flow through the cantilever. A cell with a density greater than that of the fluid appears as a positive buoyant mass, and a cell with a density less than that of the fluid appears as a negative buoyant mass (Fig. 6). This single-cell technique enables the timing and detection of density changes in biological samples as they occur in growth media.

It has a higher time resolution than current gradient-based approaches and does not involve a subsequent gradient fractionation to define the density distribution . This method may also be applied to cells transitioning to lower density by decreasing the fluid density to below that of the cells' initial density. When a sample passes through the vibrating tube sensor, its buoyant mass is recorded as a brief peak in the plot of resonance frequency vs. time (e.g., Fig 1C and 1D). Once a peak is located, the height of the peak can be measured and converted to a corresponding buoyant mass value using the sensor's point mass calibration described below.

Alternatively, a custom Python program can be used to fit the raw frequency measurements to an analytical equation of expected peak shape derived from Dohn et al. The resulting buoyant mass measurements were recorded and processed using a moving window average filter with a window size of five data points to slightly reduce noise in the plots of buoyant mass vs. time. Additional details about signal processing are provided in S6 Fig. Where mo is the absolute mass of the object, ρo is the density of the object, and ρf is the density of the fluid filling the channel. Stated in words, an object's buoyant mass is equal to its real mass minus the mass of an equivalent-volume amount of fluid.

If the object's density is greater than the fluid's density, then the object has a positive buoyant mass and its passage through the vibrating tube will be recorded as a momentary decrease in the tube's resonance frequency . If the object's density is less than the fluid's density, then the object has a negative buoyant mass and its passage through the tube will result in a momentary increase in the tube's resonance frequency . Finally, if the object's density equals the fluid's density, then the object will have zero buoyant mass and its passage through the tube will have no effect on the resonance frequency of the tube . Note that while the vibrating tube sensor is sensitive to an object's buoyant mass, it is not affected by buoyant forces because the object being measured is confined to the tube and cannot sink or float vertically. Thus, the orientation of the vibrating tube with respect to gravity has no effect on its measurements. Measurements of an object's fundamental physical properties like mass, volume, and density can offer valuable insights into the composition and state of the object.

However, many important biological samples reside in a liquid environment where it is difficult to accurately measure their physical properties. Since all objects have these physical properties, this sensor has immediate applications in a wide variety of different fields including developmental biology, toxicology, materials science, plant science, and many others. To determine the density of an irregular solid in pellet form, add approximately 40 mL of water to a clean and dry 100-mL graduated cylinder.

Place the cylinder on an analytical balance and tare. Add approximately 10 pellets, and record the new volume after the addition. The mass is only the pellets, as the rest have been tared.

Make at least two additional sets of mass and volume measurements to calculate an average value of the density. The density for zinc was measured for three different samples. Note that, since the measurements were made in a graduated cylinder, which is less precise than a volumetric flask, the density has lower degree of precision. In order to address this limitation and to measure an uninterrupted cell cycle, we continuously sampled from split cultures of elutriated G1 yeast cells that remained in media for both measurements.

Buoyant mass and volume growth rates increase during the cell cycle, and the coefficient of variation for volume is greater than that observed in buoyant mass (Figs. 5 and S2–S4). The distributions widen as the culture loses synchrony and cell size variation emerges. Although the smaller sample size prevented accurate density calculations, this approach offers a powerful means to observe growth dynamics in synchronized cell populations.

To measure the density of a sample of material, both the mass and volume of the sample must be determined. For both solids and liquids, a balance can be used to measure mass; however, methods for determining volume are different for solids and liquids. As liquids can flow and take the shapes of their containers, glassware such as a graduated cylinder or volumetric flask can be used to measure the volume of a liquid. The volume of an irregularly-shaped solid can be measured by submersion in a liquid — the difference in volume caused by addition of the solid is equal to the volume of the solid. To validate our technique with a diverse range of biological samples, we used vibrating tube sensors to monitor the mass of individual plant seeds during imbibition and germination.

Seed imbibition, or water uptake, is used in agriculture as a metric of seed health and quality . Seed germination is a change in seed metabolism when imbibition starts; germination culminates with the elongation of the embryonic axis that penetrates the seed coating. The imbibition of seeds is accompanied by a rapid leakage of cellular materials and the rate of this leakage is decreased as the tissues become hydrated . If water uptake by the seed is too rapid, the seed tissue might experience injury, and if the seed enters an anaerobic state, the seed might experience accumulation of toxic chemicals such as ethanol. Both situations can encourage undesirable seed dormancy and delay germination .

In summary, seed imbibition and germination are important phenomena in plant research, and quantitative measurements of these phenomena would be valuable in a wide range of botanical and agricultural fields. Magnesium ribbon with a thickness of 250 μm (98% pure; MiniScience Inc., Clifton, NJ) was used as a model biomaterial in our degradation rate measurement studies. Roughly 1 mm sized pieces of magnesium were cut from the ribbon. The samples were polished before measurement using 600, 800, and 1200 grit silicon carbide abrasive papers to remove the native oxide layer. Each magnesium sample was then immersed in simulated bodily fluid (phosphate-buffered saline ) with adjusted pH and passed back and forth through the vibrating tube sensor every 30 seconds until the piece had fully degraded. Flow through the sensor was controlled using the servomotor as described previously.

The resulting buoyant mass measurements are shown in Fig 4. The yeast strains used in this study are listed in Table 1. Cells were grown in YEPD at room temperature (21 ºC).

Cells were synchronized with 5 μg/mL alpha factor at 0 and 90 min, for a total arrest of 120 min, with the exception of cells synchronized by the 120-min hydroxyurea (10 mg/mL) or nocodazole (15 μg/mL) treatment. An equal volume DMSO mock-treated culture was a control for the nocodazole measurements. For cdc28-as1, cells were arrested with 1-NM-PP1 (5 μM) for 3 hr.

For the density measurements on growth-arrested cells, cells were washed via vacuum filtration at 120 min and concentrated in phosphate buffer to ∼108cells/mL. We observed no change in cell volume resulting from suspension in phosphate buffer. We compared the original sample volume distribution to that of cells collected from the SMR waste and directly observed the SMR's preferential selection of small particles. Accurate measurements of cell size are fundamental to understanding the cell cycle and to identifying cell type and cell state. The key to cell cycle control is the concentration of critical regulatory proteins, which is defined not only by expression levels, but the volume of the cell.

Furthermore, cell volume is coupled to mass and energy requirements that control cell division and survival. Changes to rates of mass and volume accumulation may be correlated with cell cycle position and can be measured as variations in cell density. The density of a substance can be used to define the substance.Water is unusual because when water freezes, its solid form is less dense than liquid water, and thus floats on top of liquid water. Raw resonance frequency data from repeated measurements of a single polyethylene microbead in water (from the right-hand distribution of measurements in Fig 1E). After zooming in to the filtered data , peaks corresponding to individual measurements of the microbead are visible.

Zooming in further on one pair of peaks shows the ∼2 millihertz height of these peaks (corresponding to a buoyant mass of ∼3.7 μg for this microbead). The peaks come in pairs because the particular vibrating tube sensor used for this measurement had a tuning-fork shape with two vibrating "U"-shaped lobes . Additional measurements of the buoyant mass of single zebrafish embryos in water, obtained using our vibrating glass tube sensor. We then hypothesized that mass measurements from vibrating tubes can give insights into the health of organisms. Previous work with the microfluidic SMR mass sensor showed that single cells undergo predictable changes in mass during normal development and reproduction .

Thus, any deviation from the normal growth trajectory can be used as a signal for abnormal development or the emergence of an illness in a cell. On the other end of the size spectrum from cells, measurements of human body weight provide some of the most fundamental insights in the health and growth of a person. We hypothesize that the same relationship between mass change and organism health applies for organisms throughout the size spectrum from cells to humans. As an object passes through the vibrating tube sensor, the shape of each resulting peak in the tube's resonance frequency is a function of the vibrational mode and amplitude of the tube.

In this work, the tubes are vibrating at their primary vibrational mode, meaning that the amplitude of vibration is highest at the tip (the bottom of the glass "U") and lowest at the base (the top of the "U"). However, as the object rounds the tip of the tube (point "b" in Fig 1B), the vibrational amplitude at the tip is the highest, so the object has a maximum effect on the tube's resonance frequency here (point "b" in Fig 1D). As the particle leaves the tube (point "c" in Fig 1B) the tube's vibrational amplitude in this region decreases again, so the resonance frequency of the tube returns to baseline (point "c" in Fig 1D). General mathematical expressions for predicting this peak shape for any vibrational mode were derived by Dohn et al.

This linkage between mass and membrane growth is central to how the cell coordinates growth with division and may have an important role in the signal for cell cycle progression. The density measurement techniques may be generalized to other cells and subcellular particles, and future technology developments aim to acquire single-cell mass and density measurements at once and with only the SMR. Because the differences in density could be artifacts of the different treatments or the cell cycle arrests, we sought to determine if cell density varied during an unperturbed cell cycle. For this purpose we measured the buoyant mass, volume, and density of G1 cells isolated by centrifugal elutriation, resuspended in media for synchronous cell cycle progression, and formaldehyde-fixed overnight.

Although fixation artificially increases cell density (Fig. 3A), the relative density throughout the time course (Fig. 4A) agrees with our previous observations and others' . Cell density was correlated with cell cycle position by comparing the cell density with the percent of budded cells (Fig. 4A, numbers in brackets). The density is relatively low in elutriated G1 cells, increases to near maximum during late G1 and S-phase entry , and then decreases through mitosis. The recorded cell density does not return to its minimum at the end of the first cell cycle because of the time course's resolution, loss of synchrony, or a possible elutriation effect on the initial selection. Buoyant mass and volume continue to increase throughout the time course as the cells grow and the population adjusts from the elutriation's size selection (Fig. 4B). The cantilever vibrates in and out of plane with an amplitude of a few hundred nanometers.

The number of single-cell measurements was maximized by adjusting the sample concentration. A more detailed description of the instrument and its operation is available in ref.12. The frequency shift as cells flow through the microchannel is position-dependent, and the maximum frequency shift, which is proportional to the recorded buoyant mass, occurs when the cell is at the cantilever tip. The frequency returns to baseline upon each cell's exit from the cantilever. Now, Karen needs to take some measurements of her ring to figure out if its density matches that of gold.

First, she uses the balance to measure the mass of the ring, which is 1.35g. To do this, Karen fills a graduated cylinder with 100 milliliters of water and then drops in the ring. Karen measures the new volume of the water, 100.5 milliliters, and then subtracts 100 milliliters. She's left with 0.5mL, which is equal to 0.5 cubic centimeters, the volume of her ring.